
myCBSEguide App
Download the app to get CBSE Sample Papers 2023-24, NCERT Solutions (Revised), Most Important Questions, Previous Year Question Bank, Mock Tests, and Detailed Notes.
Install NowNCERT Solutions for Class 9 Maths Exercise 8.1 book solutions are available in PDF format for free download. These ncert book chapter wise questions and answers are very helpful for CBSE board exam. CBSE recommends NCERT books and most of the questions in CBSE exam are asked from NCERT text books. Class 9 Maths chapter wise NCERT solution for Maths Book for all the chapters can be downloaded from our website and myCBSEguide mobile app for free.
NCERT solutions for Class 9 Maths Quadrilateral Download as PDF

NCERT Solutions for Class 9 Mathematics Quadrilaterals
1. The angles of a quadrilateral are in the ratio 3: 5: 9: 13. Find all angles of the quadrilateral.
Ans. Let in quadrilateral ABCD,  A =
A = 
 B =
B = 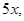
 C =
C = 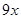 and
and  D =
D = 
Since, sum of all the angles of a quadrilateral = 

 A +
A +  B +
B +  C +
C +  D =
D = 

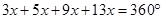

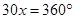


Now  A =
A = 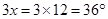
 B =
B = 
 C =
C = 
And  D =
D = 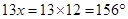
Hence angles of given quadrilateral are 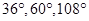 and
and 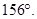
2. If the diagonals of a parallelogram are equal, show that it is a rectangle.
Ans. Given: ABCD is a parallelogram with diagonal AC = diagonal BD
To prove: ABCD is a rectangle.
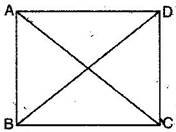
Proof: In triangles ABC and ABD,
AB = AB[Common]
AC = BD[Given]
AD = BC[opp. Sides of a  gm]
gm]

 ABC
ABC  BAD [By SSS congruency]
BAD [By SSS congruency]

 DAB =
DAB =  CBA [By C.P.C.T.] ……….(i)
CBA [By C.P.C.T.] ……….(i)
But  DAB +
DAB +  CBA =
CBA =  ……….(ii)
……….(ii)
[ AD
AD BC and AB cuts them, the sum of the interior angles of the same side of transversal is
BC and AB cuts them, the sum of the interior angles of the same side of transversal is  ]
]
From eq. (i) and (ii),
 DAB =
DAB =  CBA =
CBA = 
Hence ABCD is a rectangle.
3. Show that is diagonals of a quadrilateral bisect each other at right angles, then it is a rhombus.
Ans. Given: Let ABCD is a quadrilateral.
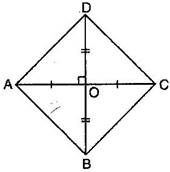
Let its diagonal AC and BD bisect each other at right angle at point O.
 OA = OC, OB = OD
OA = OC, OB = OD
And  AOB =
AOB =  BOC =
BOC =  COD =
COD =  AOD =
AOD = 
To prove: ABCD is a rhombus.
Proof: In  AOD and
AOD and  BOC,
BOC,
OA = OC[Given]
 AOD =
AOD =  BOC [Given]
BOC [Given]
OB = OD[Given]

 AOD
AOD  COB [By SAS congruency]
COB [By SAS congruency]
 AD = CB[By C.P.C.T.] ……….(i)
AD = CB[By C.P.C.T.] ……….(i)
Again, In  AOB and
AOB and  COD,
COD,
OA = OC[Given]
 AOB =
AOB =  COD [Given]
COD [Given]
OB = OD[Given]

 AOB
AOB  COD [By SAS congruency]
COD [By SAS congruency]
 AD = CB [By C.P.C.T.] ……….(ii)
AD = CB [By C.P.C.T.] ……….(ii)
Now In  AOD and
AOD and  BOC,
BOC,
OA = OC[Given]
 AOB =
AOB =  BOC [Given]
BOC [Given]
OB = OB[Common]

 AOB
AOB  COB [By SAS congruency]
COB [By SAS congruency]
 AB = BC[By C.P.C.T.] ……….(iii)
AB = BC[By C.P.C.T.] ……….(iii)
From eq. (i), (ii) and (iii),
AD = BC = CD = AB
And the diagonals of quadrilateral ABCD bisect each other at right angle.
Therefore, ABCD is a rhombus.
4. Show that the diagonals of a square are equal and bisect each other at right angles.
Ans. Given: ABCD is a square. AC and BD are its diagonals bisect each other at point O.
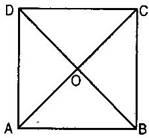
To prove: AC = BD and AC  BD at point O.
BD at point O.
Proof: In triangles ABC and BAD,
AB = AB[Common]
 ABC =
ABC =  BAD =
BAD = 
BC = AD[Sides of a square]

 ABC
ABC  BAD [By SAS congruency]
BAD [By SAS congruency]
 AC = BD[By C.P.C.T.]Hence proved.
AC = BD[By C.P.C.T.]Hence proved.
Now in triangles AOB and AOD,
AO = AO[Common]
AB = AD[Sides of a square]
OB = OD[Diagonals of a square bisect each other]

 AOB
AOB  AOD [By SSS congruency]
AOD [By SSS congruency]
 AOB =
AOB =  AOD [By C.P.C.T.]
AOD [By C.P.C.T.]
But  AOB +
AOB +  AOD =
AOD =  [Linear pair]
[Linear pair]

 AOB =
AOB =  AOD =
AOD = 
 OA
OA  BD or AC
BD or AC  BD Hence proved.
BD Hence proved.
NCERT Solutions for Class 9 Maths Exercise 8.1
5. Show that if the diagonals of a quadrilateral are equal and bisect each other at right angles, then it is a square.
Ans. Let ABCD be a quadrilateral in which equal diagonals AC and BD bisect each other at right angle at point O.
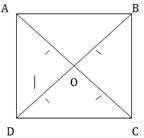
We haveAC = BD and OA = OC……….(i)
And OB = OD……….(ii)
Now OA + OC = OB + OD
 OC + OC = OB + OB [Using (i) & (ii)]
OC + OC = OB + OB [Using (i) & (ii)]
 2OC = 2OB
2OC = 2OB
 OC = OB……….(iii)
OC = OB……….(iii)
From eq. (i), (ii) and (iii), we get, OA = OB = OC = OD ……….(iv)
Now in  AOB and
AOB and  COD,
COD,
OA = OD [proved]
 AOB =
AOB =  COD[vertically opposite angles]
COD[vertically opposite angles]
OB = OC [proved]

 AOB
AOB  DOC[By SAS congruency]
DOC[By SAS congruency]
 AB = DC [By C.P.C.T.]……….(v)
AB = DC [By C.P.C.T.]……….(v)
Similarly,  BOC
BOC  AOD [By SAS congruency]
AOD [By SAS congruency]
 BC = AD [By C.P.C.T.]……….(vi)
BC = AD [By C.P.C.T.]……….(vi)
From eq. (v) and (vi), it is concluded that ABCD is a parallelogram because opposite sides of a quadrilateral are equal.
Now in  ABC and
ABC and  BAD,
BAD,
AB = BA [Common]
BC = AD [proved above]
AC = BD [Given]

 ABC
ABC  BAD[By SSS congruency]
BAD[By SSS congruency]

 ABC =
ABC =  BAD[By C.P.C.T.]……….(vii)
BAD[By C.P.C.T.]……….(vii)
But  ABC +
ABC +  BAD =
BAD =  [ABCD is a parallelogram]……….(viii)
[ABCD is a parallelogram]……….(viii)
 AD
AD  BC and AB is a transversal.
BC and AB is a transversal.

 ABC +
ABC +  ABC =
ABC =  [Using eq. (vii) and (viii)]
[Using eq. (vii) and (viii)]
 2
2 ABC =
ABC = 

 ABC =
ABC = 

 ABC =
ABC =  BAD =
BAD = ……….(ix)
……….(ix)
Opposite angles of a parallelogram are equal.
But  ABC =
ABC =  BAD =
BAD =

 ABC =
ABC =  ADC =
ADC =  ……….(x)
……….(x)

 BAD =
BAD =  BDC =
BDC = ……….(xi)
……….(xi)
From eq. (x) and (xi), we get
 ABC =
ABC =  ADC =
ADC =  BAD =
BAD =  BDC =
BDC = ……….(xii)
……….(xii)
Now in  AOB and
AOB and  BOC,
BOC,
OA = OC [Given]
 AOB =
AOB =  BOC =
BOC =  [Given]
[Given]
OB = OB [Common]

 AOB
AOB  COB[By SAS congruency]
COB[By SAS congruency]
 AB = BC……….(xiii)
AB = BC……….(xiii)
From eq. (v), (vi) and (xiii), we get,
AB = BC = CD = AD ……….(xiv)
Now, from eq. (xii) and (xiv), we have a quadrilateral whose equal diagonals bisect each other at right angle.
Also sides are equal make an angle of  with each other.
with each other.
 ABCD is a square.
ABCD is a square.
NCERT Solutions for Class 9 Maths Exercise 8.1
6. Diagonal AC of a parallelogram ABCD bisects  A (See figure). Show that:
A (See figure). Show that:
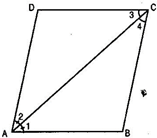
(i) It bisects  C also.
C also.
(ii) ABCD is a rhombus.
Ans. Diagonal AC bisects  A of the parallelogram ABCD.
A of the parallelogram ABCD.
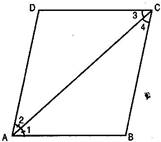
(i) Since AB  DC and AC intersects them.
DC and AC intersects them.

 1 =
1 =  3 [Alternate angles] ……….(i)
3 [Alternate angles] ……….(i)
Similarly  2 =
2 =  4 ……….(ii)
4 ……….(ii)
But  1 =
1 =  2 [Given]……….(iii)
2 [Given]……….(iii)

 3 =
3 =  4[Using eq. (i), (ii) and (iii)]
4[Using eq. (i), (ii) and (iii)]
Thus AC bisects  C.
C.
(ii)  2 =
2 =  3 =
3 =  4 =
4 =  1
1
 AD = CD[Sides opposite to equal angles]
AD = CD[Sides opposite to equal angles]
 AB = CD = AD = BC
AB = CD = AD = BC
Hence ABCD is a rhombus.
NCERT Solutions for Class 9 Maths Exercise 8.1
7. ABCD is a rhombus. Show that the diagonal AC bisects  A as well as
A as well as  C and diagonal BD bisects
C and diagonal BD bisects  B as well as
B as well as  D.
D.
Ans. ABCD is a rhombus. Therefore, AB = BC = CD = AD
Let O be the point of bisection of diagonals.
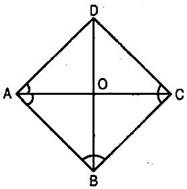
 OA = OC and OB = OD
OA = OC and OB = OD
In  AOB and
AOB and  AOD,
AOD,
OA = OA [Common]
AB = AD [Equal sides of rhombus]
OB = OD (diagonals of rhombus bisect each other]

 AOB
AOB  AOD [By SSS congruency]
AOD [By SSS congruency]

 OAD =
OAD =  OAB [By C.P.C.T.]
OAB [By C.P.C.T.]
 OA bisects
OA bisects  A ……….(i)
A ……….(i)
Similarly,  BOC
BOC  DOC [By SSS congruency]
DOC [By SSS congruency]

 OCB =
OCB =  OCD[By C.P.C.T.]
OCD[By C.P.C.T.]
 OC bisects
OC bisects  C ……….(ii)
C ……….(ii)
From eq. (i) and (ii), we can say that diagonal AC bisects  A and
A and  C.
C.
Now in  AOB and
AOB and  BOC,
BOC,
OB = OB [Common]
AB = BC [Equal sides of rhombus]
OA = OC (diagonals of rhombus bisect each other]

 AOB
AOB  COB [By SSS congruency]
COB [By SSS congruency]

 OBA =
OBA =  OBC [By C.P.C.T.]
OBC [By C.P.C.T.]
 OB bisects
OB bisects  B ……….(iii)
B ……….(iii)
Similarly,  AOD
AOD  COD [By SSS congruency]
COD [By SSS congruency]

 ODA =
ODA =  ODC[By C.P.C.T.]
ODC[By C.P.C.T.]
 BD bisects
BD bisects  D ……….(iv)
D ……….(iv)
From eq. (iii) and (iv), we can say that diagonal BD bisects  B and
B and  D.
D.
NCERT Solutions for Class 9 Maths Exercise 8.1
8. ABCD is a rectangle in which diagonal AC bisects  A as well as
A as well as  C. Show that:
C. Show that:
(i) ABCD is a square.
(ii) Diagonal BD bisects both  B as well as
B as well as  D.
D.
Ans. ABCD is a rectangle. Therefore AB = DC……….(i)
And BC = AD
Also  A =
A =  B =
B =  C =
C =  D =
D = 

(i) In  ABC and
ABC and  ADC
ADC
 1 =
1 =  2 and
2 and  3 =
3 =  4
4
[AC bisects  A and
A and  C (given)]
C (given)]
AC = AC [Common]

 ABC
ABC  ADC[By ASA congruency]
ADC[By ASA congruency]
 AB = AD ……….(ii)
AB = AD ……….(ii)
From eq. (i) and (ii), AB = BC = CD = AD
Hence ABCD is a square.

(ii) In  ABC and
ABC and  ADC
ADC
AB = BA [Since ABCD is a square]
AD = DC [Since ABCD is a square]
BD = BD [Common]

 ABD
ABD  CBD [By SSS congruency]
CBD [By SSS congruency]

 ABD =
ABD =  CBD [By C.P.C.T.]……….(iii)
CBD [By C.P.C.T.]……….(iii)
And  ADB =
ADB =  CDB[By C.P.C.T.]……….(iv)
CDB[By C.P.C.T.]……….(iv)
From eq. (iii) and (iv), it is clear that diagonal BD bisects both  B and
B and  D.
D.
NCERT Solutions for Class 9 Maths Exercise 8.1
9. In parallelogram ABCD, two points P and Q are taken on diagonal BD such that DP = BQ (See figure). Show that:
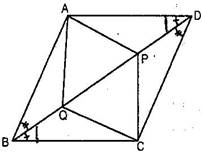
(i)  APD
APD  CQB
CQB
(ii) AP = CQ
(iii)  AQB
AQB  CPD
CPD
(iv) AQ = CP
(v) APCQ is a parallelogram.
Ans. (i) In  APD and
APD and  CQB,
CQB,
DP = BQ[Given]
 ADP =
ADP =  QBC [Alternate angles (AD
QBC [Alternate angles (AD BC and BD is transversal)]
BC and BD is transversal)]
AD = CB [Opposite sides of parallelogram]

 APD
APD  CQB [By SAS congruency]
CQB [By SAS congruency]
(ii) Since  APD
APD  CQB
CQB
 AP = CQ[By C.P.C.T.]
AP = CQ[By C.P.C.T.]
(iii) In  AQB and
AQB and  CPD,
CPD,
BQ = DP[Given]
 ABQ =
ABQ =  PDC [Alternate angles (AB
PDC [Alternate angles (AB CD and BD is transversal)]
CD and BD is transversal)]
AB = CD[Opposite sides of parallelogram]

 AQB
AQB  CPD [By SAS congruency]
CPD [By SAS congruency]
(iv) Since  AQB
AQB  CPD
CPD
 AQ = CP[By C.P.C.T.]
AQ = CP[By C.P.C.T.]
(v) In quadrilateral APCQ,
AP = CQ[proved in part (i)]
AQ = CP[proved in part (iv)]
Since opposite sides of quadrilateral APCQ are equal.
Hence APCQ is a parallelogram.
NCERT Solutions for Class 9 Maths Exercise 8.1
10. ABCD is a parallelogram and AP and CQ are the perpendiculars from vertices A and C on its diagonal BD (See figure). Show that:
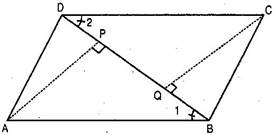
(i)  APB
APB  CQD
CQD
(ii) AP = CQ
Ans. Given: ABCD is a parallelogram. AP  BD and CQ
BD and CQ 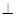 BD
BD
To prove: (i)  APB
APB  CQD (ii) AP = CQ
CQD (ii) AP = CQ
Proof: (i) In  APB and
APB and  CQD,
CQD,
 1 =
1 =  2[Alternate interior angles]
2[Alternate interior angles]
AB = CD[Opposite sides of a parallelogram are equal]
 APB =
APB =  CQD =
CQD = 

 APB
APB  CQD [By ASA Congruency]
CQD [By ASA Congruency]
(ii) Since  APB
APB  CQD
CQD
 AP = CQ [By C.P.C.T.]
AP = CQ [By C.P.C.T.]
NCERT Solutions for Class 9 Maths Exercise 8.1
11. An  ABC and
ABC and  DEF, AB = DE, AB
DEF, AB = DE, AB  DE, BC = EF and BC
DE, BC = EF and BC  EF. Vertices A, B and C are joined to vertices D, E and F respectively (See figure). Show that:
EF. Vertices A, B and C are joined to vertices D, E and F respectively (See figure). Show that:
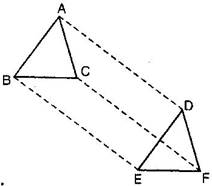
(i) Quadrilateral ABED is a parallelogram.
(ii) Quadrilateral BEFC is a parallelogram.
(iii) AD  CF and AD = CF
CF and AD = CF
(iv) Quadrilateral ACFD is a parallelogram.
(v) AC = DF
(vi)  ABC
ABC  DEF
DEF
Ans. (i) In  ABC and
ABC and  DEF
DEF
AB = DE[Given]
And AB  DE[Given]
DE[Given]
 ABED is a parallelogram.
ABED is a parallelogram.
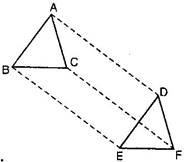
(ii) In  ABC and
ABC and  DEF
DEF
BC = EF[Given]
And BC  EF[Given]
EF[Given]
 BEFC is a parallelogram.
BEFC is a parallelogram.
(iii) As ABED is a parallelogram.
 AD
AD  BE and AD = BE ……….(i)
BE and AD = BE ……….(i)
Also BEFC is a parallelogram.
 CF
CF  BE and CF = BE……….(ii)
BE and CF = BE……….(ii)
From (i) and (ii), we get
 AD
AD  CF and AD = CF
CF and AD = CF
(iv) As AD  CF and AD = CF
CF and AD = CF
 ACFD is a parallelogram.
ACFD is a parallelogram.
(v) As ACFD is a parallelogram.
 AC = DF
AC = DF
(vi) In  ABC and
ABC and  DEF,
DEF,
AB = DE [Given]
BC = EF [Given]
AC = DF [Proved]

 ABC
ABC 
 DEF[By SSS congruency]
DEF[By SSS congruency]
NCERT Solutions for Class 9 Maths Exercise 8.1
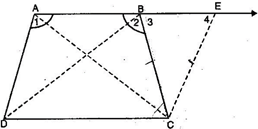
12. ABCD is a trapezium in which AB  CD and AD = BC (See figure). Show that:
CD and AD = BC (See figure). Show that:
(i)  A =
A =  B
B
(ii)  C =
C =  D
D
(iii)  ABC
ABC  BAD
BAD
(iv) Diagonal AC = Diagonal BD
Ans. Given: ABCD is a trapezium.
AB  CD and AD = BC
CD and AD = BC
To prove: (i)  A =
A =  B
B
(ii)  C =
C =  D
D
(iii)  ABC
ABC 
 BAD
BAD
(iv) Diag. AC = Diag. BD
Construction: Draw CE  AD and extend
AD and extend
AB to intersect CE at E.
Proof: (i) As AECD is a parallelogram.[By construction]
 AD = EC
AD = EC
But AD = BC [Given]
 BC = EC
BC = EC

 3 =
3 =  4 [Angles opposite to equal sides are equal]
4 [Angles opposite to equal sides are equal]
Now  1 +
1 +  4 =
4 = 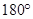 [Interior angles]
[Interior angles]
And  2 +
2 +  3 =
3 = 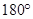 [Linear pair]
[Linear pair]

 1 +
1 +  4 =
4 =  2 +
2 +  3
3

 1 =
1 =  2 [
2 [
 3 =
3 =  4 ]
4 ]

 A =
A =  B
B
(ii)  3 =
3 =  C[Alternate interior angles]
C[Alternate interior angles]
And  D =
D =  4 [Opposite angles of a parallelogram]
4 [Opposite angles of a parallelogram]
But  3 =
3 =  4 [
4 [ BCE is an isosceles triangle]
BCE is an isosceles triangle]

 C =
C =  D
D
(iii) In  ABC and
ABC and  BAD,
BAD,
AB = AB [Common]
 1 =
1 =  2 [Proved]
2 [Proved]
AD = BC [Given]

 ABC
ABC  BAD[By SAS congruency]
BAD[By SAS congruency]
(iv) We had observed that,

 ABC
ABC  BAD
BAD
 AC = BD [By C.P.C.T.]
AC = BD [By C.P.C.T.]
NCERT Solutions for Class 9 Maths Exercise 8.1
NCERT Solutions for Class 9 Maths PDF (Download) Free from myCBSEguide app and myCBSEguide website. Ncert solution class 9 Maths includes text book solutions from Mathematics Book. NCERT Solutions for CBSE Class 9 Maths have total 15 chapters. 9 Maths NCERT Solutions in PDF for free Download on our website. Ncert Maths class 9 solutions PDF and Maths ncert class 9 PDF solutions with latest modifications and as per the latest CBSE syllabus are only available in myCBSEguide.
CBSE app for Class 9
To download NCERT Solutions for Class 9 Maths, Computer Science, Home Science,Hindi ,English, Social Science do check myCBSEguide app or website. myCBSEguide provides sample papers with solution, test papers for chapter-wise practice, NCERT solutions, NCERT Exemplar solutions, quick revision notes for ready reference, CBSE guess papers and CBSE important question papers. Sample Paper all are made available through the best app for CBSE students and myCBSEguide website.

Test Generator
Create question paper PDF and online tests with your own name & logo in minutes.
Create Now
myCBSEguide
Question Bank, Mock Tests, Exam Papers, NCERT Solutions, Sample Papers, Notes
Install Now
Please a search box on this website so that the user can search his question and he could its solution.